IB Physics Imaging option: Magnification of optical instruments
Take note of sign conventions!
Magnifying glasses
A single convex lens can be used as a magnifying glass.
If an object \(O\) is placed within the focal point \(F\) of a convex lens, a virtual image \(I\) will be formed.
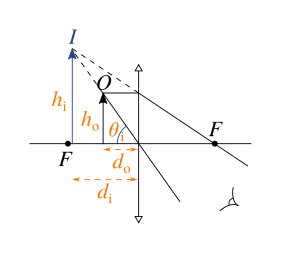
The linear magnification \(m\) of the magnifying glass is the ratio of the height of the image to the height of the object. Using similar triangles, \(m = \frac{h_\text{i}}{h_\text{o}} = -\frac{d_\text{i}}{d_\text{o}}\). This is the formula given in the IB data booklet.
The angular magnification \(M\) is the ratio of the angle subtended by the image using the magnifying glass, to the angle subtended by the object using the unaided eye at the near point (\(D \approx 25\text{ cm}\)). (Some sources say the angular magnification is the ratio of the tangent of these two angles, but since the angles involved are small, \(\tan θ \approx θ\) so the formula is unchanged.)
Let the angle subtended by the object using the unaided eye at the near point be \(θ_\text{o}\). Note that \(\tan θ_\text{o} = \frac{h_\text{o}}{D}\), but since the angles involved are small, \(\tan θ_\text{o} \approx θ_\text{o}\), hence \(θ_\text{o} = \frac{h_\text{o}}{D}\). Similarly, the angle subtended by the final image using the magnifying glass is \(θ_\text{i} = \frac{h_\text{i}}{-d_\text{i}}\).
Thus the angular magnification of the magnifying glass is \(M = \frac{θ_\text{i}}{θ_\text{o}} = \frac{\frac{h_\text{i}}{-d_\text{i}}}{\frac{h_\text{o}}{D}} = -\frac{h_\text{i}}{h_\text{o}} \cdot \frac{D}{d_\text{i}} = \frac{d_\text{i}}{d_\text{o}} \cdot \frac{D}{d_\text{i}} = \frac{D}{d_\text{o}}\).
If the image is formed at the near point, \(d_\text{i} = -D\), but by the lens equation, \(\frac{1}{d_\text{i}} + \frac{1}{d_\text{o}} = \frac{1}{f}\), so \(\frac{1}{d_\text{o}} = \frac{1}{f} - \frac{1}{d_\text{i}} = \frac{1}{f} + \frac{1}{D}\), so \(M = D\left(\frac{1}{f} + \frac{1}{D}\right) = \frac{D}{f} + 1\). This is the formula given in the IB data booklet.
Returning to the formula \(M = \frac{D}{d_\text{o}}\), note that when the image is formed at the near point, \(d_\text{i} = -D\), therefore \(M = -\frac{d_\text{i}}{d_\text{o}} = m\). Thus the linear and angular magnifications are equal!
The other case of note is when the image is formed at infinity. In this case, the object must be placed at the focal point, so \(d_\text{o} = f\), so \(M = \frac{D}{d_\text{o}} = \frac{D}{f}\). This is the formula given in the IB data booklet.
Optical astronomical refracting telescopes
An astronomial refracting telescope consists of a long-focal-length objective lens and a short-focal-length eyepiece lens, to magnify objects located a long way away.
Suppose we have an object \(O\), and assume for simplicity that the object is aligned relative to the telescope as shown in the diagram below: (most definitely not to scale!)
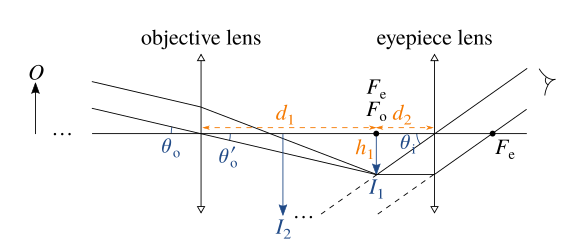
Rays from the top of the distant object approach the objective lens and are focused to form an intermediate object \(I_1\). \(I_1\) becomes the object of the eyepiece lens, and forms a final object \(I_2\).
Apparently, when the IB asks for the ‘magnification’ of a telescope, they mean the angular magnification. (Working backwards from SPEC/HP3 Q12.)
Angular magnification
The angular magnification of the telescope is the ratio of the angle subtended by the final image using the telescope, to the angle subtended by the object using the unaided eye.
The angle subtended by the object using the unaided eye is \(θ_\text{o} = -θ'_\text{o}\). Note that \(\tan θ'_\text{o} = \frac{h_1}{d_1}\), but as before, \(\tan θ'_\text{o} \approx θ'_\text{o}\), hence \(θ_\text{o} = -\frac{h_1}{d_1}\). Similarly, the angle subtended by the final image using the telescope is \(θ_\text{i} = \frac{h_1}{d_2}\).
Thus the angular magnification of the telescope is \(M = \frac{θ_\text{i}}{θ_\text{o}} = \frac{\frac{h_1}{d_2}}{-\frac{h_1}{d_1}} = -\frac{d_1}{d_2}\). If the object is not at infinity, or the telescope is not at normal adjustment, this is the angular magnification formula, and use of the lens equation may be necessary to determine the angular magnification.
However, for a distant object, the rays from the object approach effectively parallel, and so are brought to focus at the focal point of the objective lens \(F_\text{o}\), hence \(d_1 = f_\text{o}\). Furthermore, for a telescope at normal adjustment (for a ‘relaxed eye’), the final image is produced at infinity, hence the intermediate image must also be at the focal point of the eyepiece lens \(F_\text{e}\), hence \(d_2 = f_\text{e}\). Thus \(M = -\frac{f_\text{o}}{f_\text{e}}\). This is the formula in the IB data booklet.
Note also that in this situation, the separation of the lenses is \(f_\text{o} + f_\text{e}\).
Example 1: What is the magnification of an astronomical telescope whose objective lens has a focal length of 76 cm and whose eyepiece has a focal length of 2.8 cm? What is the overall length of the telescope when adjusted for use by the relaxed eye? (Giancoli, 6th ed, chapter 25, question 29)
Solution: \(M = \frac{f_\text{o}}{f_\text{e}} = \frac{76\text{ cm}}{2.8\text{ cm}} \approx 27\). \(L = f_\text{o} + f_\text{e} = 76\text{ cm} + 2.8\text{ cm} \approx 79\text{ cm}\).
Example 2: A 170× astronomical telescope is adjusted for a relaxed eye when the two lenses are 1.25 m apart. What is the focal length of each lens? (Giancoli, 6th ed, chapter 25, question 37)
Solution: \(L = f_\text{o} + f_\text{e} \Rightarrow f_\text{o} = L - f_\text{e}\). \(M = \frac{f_\text{o}}{f_\text{e}} = \frac{L - f_\text{e}}{f_\text{e}} = \frac{L}{f_\text{e}} - 1 \Rightarrow f_\text{e} = \frac{L}{M + 1} = \frac{1.25\text{ m}}{170 + 1} \approx 7.3\text{ mm}\). \(f_\text{o} = L - f_\text{e} = 1.25\text{ m} - 7.3\text{ mm} = 1.24\text{ m}\).
Optical compound microscopes
A compound microscope consists of a very-short-focal-length objective lens and a short-focal-length eyepiece lens, to magnify small objects located just outside the focal point of the objective lens.
Suppose we have an object \(O\), and assume for simplicity that the object is aligned relative to the microscope as shown in the diagram below:
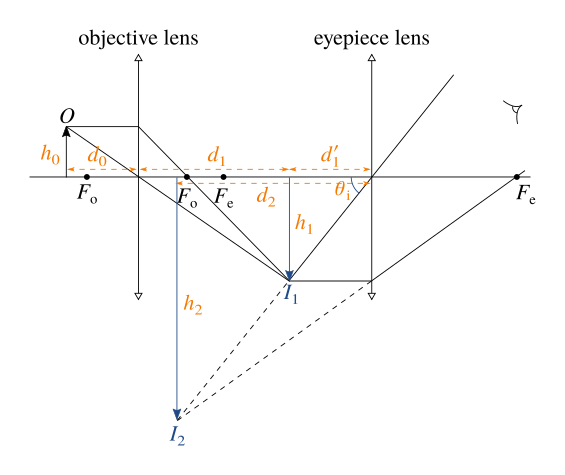
Rays from the top of the object approach the objective lens and are focused to form an intermediate object \(I_1\). \(I_1\) becomes the object of the eyepiece lens, and forms a final object \(I_2\).
Apparently, when the IB asks for the ‘magnification’ of a microscope, they mean the linear magnification. (Working backwards from M10/HP3/TZ2 G2 and M06/HP3/TZ2 H3.)
Linear magnification
The linear magnification of the microscope is the ratio of the height of the final image to the height of the object. \(m = \frac{h_2}{h_0} = \frac{h_1}{h_0} \cdot \frac{h_2}{h_1} = m_\text{o}m_\text{e}\). Thus the total linear magnification of the microscope is the product of the linear magnifications of the objective and eyepiece lenses.
In terms of distances, \(m = \frac{d_1}{d_0} \cdot \frac{d_2}{d'_1}\).
Angular magnification
The angular magnification of the microscope is the ratio of the angle subtended by the final image using the microscope, to the angle subtended by the object using the unaided eye at the near point.
Let the angle subtended by the object using the unaided eye be \(θ_\text{o}\). Note that \(\tan θ_\text{o} = \frac{h_0}{D}\), but as before, \(\tan θ_\text{o} \approx θ_\text{o}\), hence \(θ_\text{o} = \frac{h_0}{D}\). Similarly, the angle subtended by the final image using the microscope is \(θ_\text{i} = \frac{h_1}{d'_1}\).
Thus the angular magnification of the microscope is \(M = \frac{θ_\text{i}}{θ_\text{o}} = \frac{\frac{h_1}{d'_1}}{\frac{h_0}{D}} = \frac{h_1}{h_0} \cdot \frac{D}{d'_1}\). Recall from earlier that the angular magnification of a lens is \(\frac{D}{d_\text{o}}\). Thus \(M = m_\text{o}M_\text{e}\).
If the microscope is at normal adjustment, and the image is formed at the near point, recall further than \(M_\text{e} = m_\text{e}\), hence \(M = m_\text{o}m_\text{e} = m\).
Example 1: The eyepiece of a compound microscope has a focal length of 2.70 cm, and the objective lens has \(f\) = 0.740 cm. If an object is placed 0.790 cm from the objective lens, calculate the total magnification when the microscope is at normal adjustment. (Adapted from Giancoli, 6th ed, chapter 25, question 45)
Solution: \(\frac{1}{d_0} + \frac{1}{d_1} = \frac{1}{f_\text{o}} \Rightarrow \frac{1}{d_1} = \frac{1}{f_\text{o}} - \frac{1}{d_0} = \frac{1}{0.740\text{ cm}} - \frac{1}{0.790\text{ cm}} \Rightarrow d_1 \approx 10\text{ cm}\). \(m = M = m_\text{o}M_\text{e} = -\frac{d_1}{d_0} \cdot \left(\frac{D}{f_\text{e}} + 1\right) = -\frac{10\text{ cm}}{0.790\text{ cm}} \cdot \left(\frac{25\text{ cm}}{2.70\text{ cm}} + 1\right) \approx -200\) (\(-152\) to 3 s.f.)
References
A.K.A, ‘You want my name? It's “magnification”.’ ‘Okay. What magnification? What magnification?!’
SPEC/HP3 Q13: \(\text{magnification} = m_\text{o}M_\text{e}\). (normal adjustment. \(\frac{D}{f} + 1\) used.)
M14/HP3/TZ1 Q10: \(m = m_\text{o}M_\text{e}\) (!!) or \(\frac{h_\text{i}}{h_\text{o}}\). (normal adjustment stated. \(D\) taken to be 25 cm.)
N13/HP3 G8: \(M = M_\text{o}M_\text{e}\) (???). (\(M_\text{o}\) given. \(D\) taken to be 25.1 cm for \(M_\text{e}\). After some discussion, I assume this is a mistake, and the formula should be \(m = m_\text{o}m_\text{e}\).)
N12/HP3 G2: \(\text{magnification} = m_\text{o}M_\text{e}\). (normal adjustment stated. \(D\) given.)
M10/HP3/TZ2 G2: \(\text{magnification} = m_\text{o}m_\text{e}\) or \(m_\text{o} \left(\frac{d_\text{i}}{f} + 1\right)\). (not at normal adjustment.)
N07/HP3 H3: \(m = m_\text{o}m_\text{e}\).
M06/HP3/TZ2 H3: \(\text{magnification} = m_\text{o}m_\text{e}\).